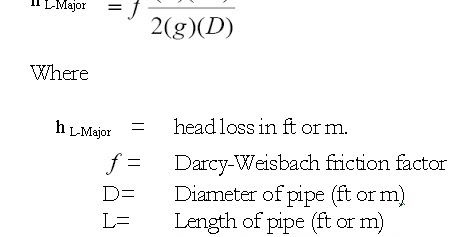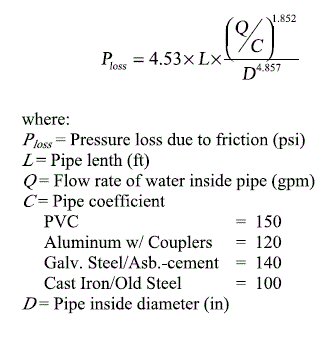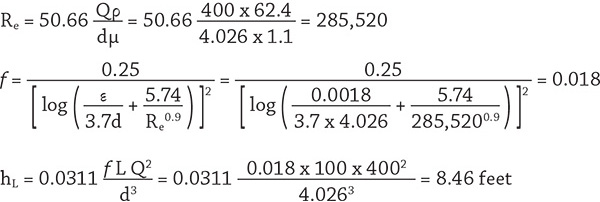Head Loss In Pipe Equation

Water pipes head loss calculator app.

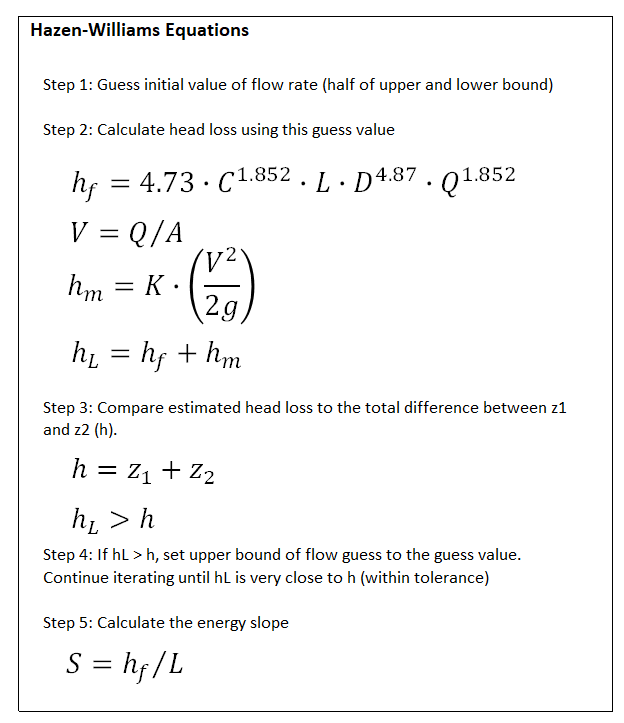
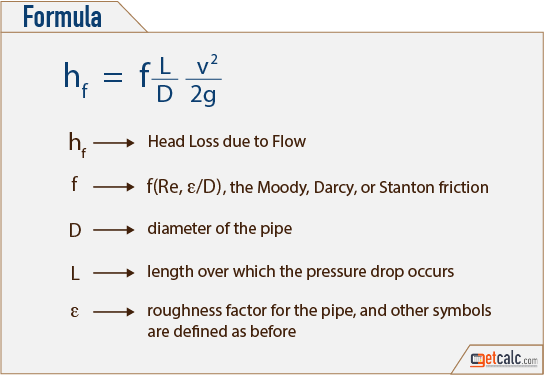
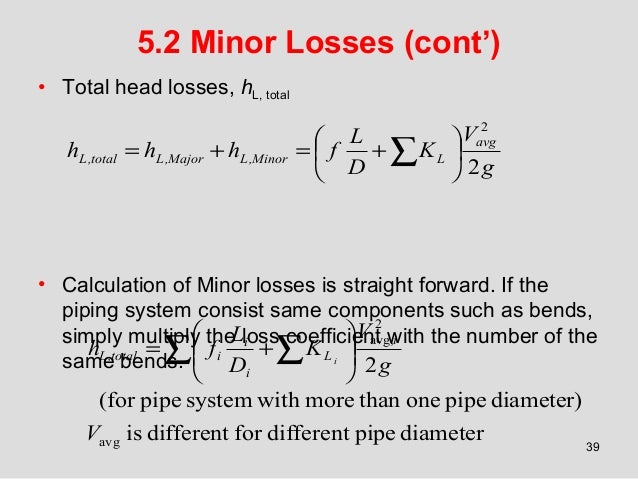
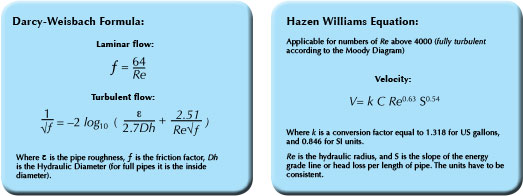
Head loss in pipe equation. The total loss can be represented by. Head loss f l v 2 d. The head loss is inversely proportional to the diameter of the pipe. For most pipe runs there will be multiple minor loss elements connected by sections of straight pipe.

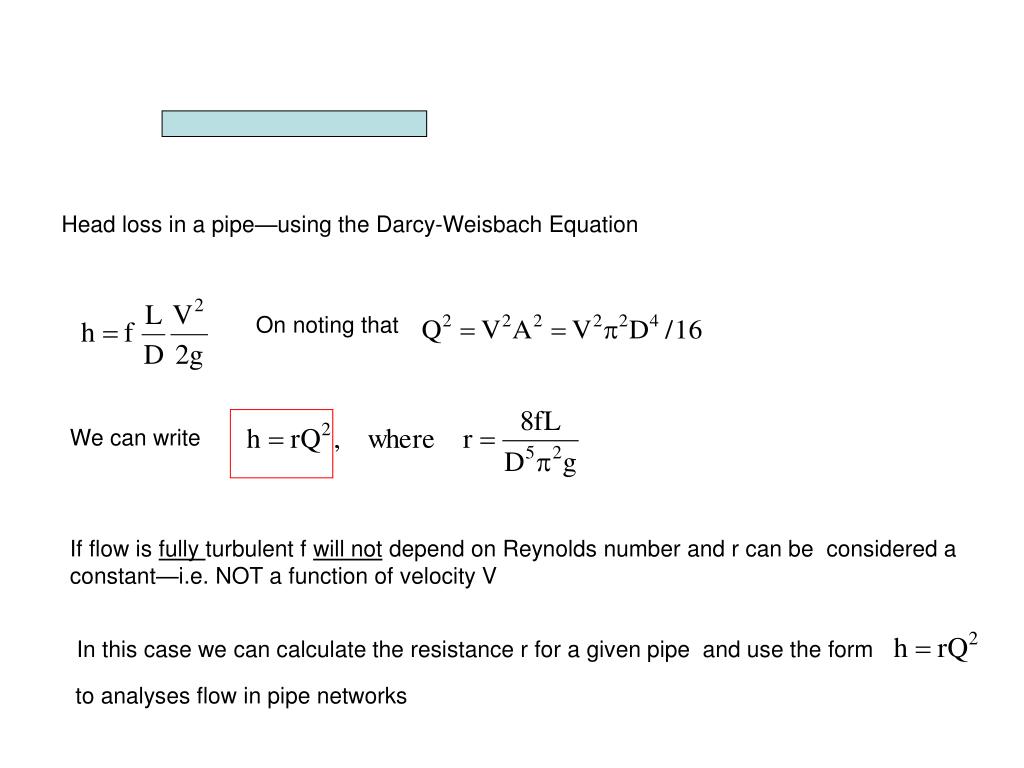
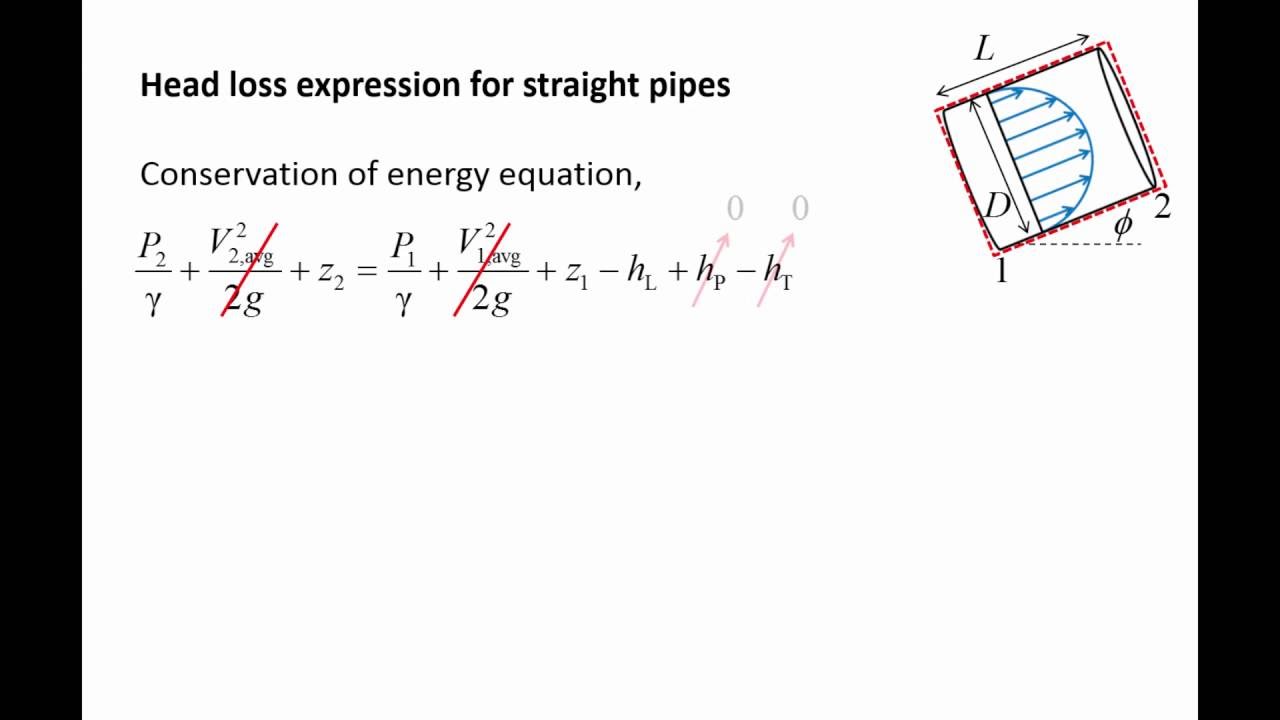
Head loss calculations bernoulli and pipe flow. Minor losses must be included in the head loss term in the energy equation. The head loss is inversely proportional to the diameter of the pipe. By observation the major head loss is roughly proportional to the square of the flow rate in most engineering flows fully developed turbulent pipe flow.

H l f l v 2 d. The head loss for 100 ft pipe can be calculated as. It takes something to move over a rough surface 2 pipe flow. H 30ft h 100ft 30 ft 100 ft 9 30 ft 100 ft 2 7 ft h 2 o.

H 100ft 0 2083 100 140 1 852 200 gal min 1 852 3 048 in 4 8655 9 ft h 2 o 100 ft pipe. The head loss for fluid flow is directly proportional to the length of pipe the square of the fluid velocity and a term accounting for fluid friction called the friction factor. The most common equation used to calculate major head losses in a tube or duct is the darcy weisbach equation head loss form. H l head loss in equation 1 α represents the energy coefficient.

G is the local acceleration due to gravity m s 2. When fluid flows inside a pipeline friction occurs between the moving fluid and the stationary. Head loss general equation. The head loss for fluid flow is directly proportional to the length of pipe the square of the fluid velocity and a term accounting for fluid friction called the friction factor.

V is the velocity within the pipe g is the acceleration due to gravity f is the coefficient of friction. Where l is the pipe length m. The bernoulli equation that we worked with was a bit simplistic in the way it looked at a fluid system. The energy coefficient is 1 when the flow is developed.

Related mobile app from the engineering toolbox. Calculating head loss in a pipeline head loss in a pipeline. However if the flow is undeveloped the energy coefficient will be less than 1. For circular sections this equals the internal diameter of the pipe m.

The head loss for 30 ft pipe can be calculated. δh is the head loss due to pipe friction over the given length of pipe si units. Often the construction material limits the available pipe sizes and schedules. All real systems that are in motion suffer from some type of loss due to friction.